Wardowski conditions to the coincidence problem

1. Introduction

Let X, Y be two nonempty sets and let T, S : X → Y be two arbitrary mappings. The coincidence problem determined by the mappings T and S consists in

$\begin{array}{cc}\text{Find}p\in X\text{such}\text{that}Tp=Sp.& (1)\end{array}$

Quite often to solve problem (1), we have to assume that Y is a complete metric space, and T, S : X → Y are two mappings satisfying some type of contractivity, for instance see [1–5]. Some nonlinear problems arising from many areas of applied sciences can be formulated, from a mathematical point of view, as a coincidence problem (see, [1–3, 6, 7] and references within).

Once the existence of a solution to problem (1) is known, a central question consists to study if there exists an approximating sequence (x_n) ⊆ X generated by an iterative procedure f(T, S, x_n) such that the sequence (x_n) converges to the coincidence point of T and S. Jungck [8] introduced the following iterative scheme: given x₁ ∈ X, there exists a sequence (x_n) in X such that Tx_n+1 = Sx_n. This procedure becomes the Picard iteration when X = Y and T = I_d, where I_d is the identity map on X. In Jungck [8], the author proved that if (X, d) and (Y, ρ) are two complete metric spaces and T and S satisfy both that S(X) ⊆ T(X) and that for every x, y ∈ X the inequality d(Sx, Sy) ≤ κ d(Tx, Ty), with 0 ≤ κ < 1 holds, then (x_n) converges to the unique coincidence point of T and S. Later, this type of convergence results were generalized for more general classes of contractive type mappings, see [6, 7, 9, 10] (to see another type of iterative schemes we can quote [10, 11]).

Since it is well known that the existence of a solution to problem (1) is, under appropriate conditions, equivalent to the existence of a fixed point for a certain mapping. In this article, we will use the Wardowski fixed point theorem [12] in order to show that problem (1) has a unique solution and that the Picard-Jungck iterative scheme converges to the unique coincidence point, moreover a rate of convergence for this scheme will also be given. Finally, we will apply these results to a general second order differential equation.

2. Notations and Preliminaries

Throughout this article ℝ₊ and ℕ will denote the set of all non-negative real numbers and the set of all positive integers respectively.

Definition 2.1. Let X and Y be two nonempty sets and T, S:X → Y two mappings. If there exists x ∈ X such that Sx = Tx then x is said to be a coincidence point of S and T.

Definition 2.2. Let S and T be two self-mappings of a nonempty set X. The pair of mappings S and T is said to be weakly compatible if they commute at their coincidence points, that is, TSx = STx whenever Tx = Sx.

The following straightforward result states a relationship between coincidence points and common fixed points of two weakly compatible mappings, see Proposition 1.4 in Abbas and Jungck [9].

Lemma 2.1. Let S and T be weakly compatible self-mappings of a nonempty set X. If S and T have a unique coincidence point x, then x is the unique common fixed point of S and T.

Given k ∈ (0, 1), by ${F}$_k denote the set of all strictly increasing real functions f:(0, ∞) → ℝ satisfying the following conditions:

(F₁) For each sequence {α_n}_n∈ℕ of positive numbers, $\underset{n\to \infty }{\lim }{\alpha }_n=0\iff \underset{n\to \infty }{\lim }f({\alpha }_n)=-\infty .$

(F₂) $\underset{\alpha \to 0^{+}}{\lim }{\alpha }^{k}f(\alpha )=0$.

Definition 2.3. Let (X, d) be a complete metric space. A mapping T : X → X is said to be an F-contraction if there exist τ > 0 and f ∈ ${F}$_k such that, for all x, y ∈ X,

$\begin{array}{cc}d(x,y)>0\Rightarrow \tau +f(d(x,y))\le f(d(Tx,Ty)).& (2)\end{array}$

The following result will be the key in the proof of our results. This result was proved by Wardowski [12].

Theorem 2.1. [[12]] Let (X, d) be a complete metric space and let T : X → X be an F-contraction. Then T has a unique fixed point x* ∈ X and for every x₀ ∈ X the sequence ${\left\{T^n(x_0)\right\}}_{n\in \mathbb{N}}$ is convergent to x*.

3. Main results

3.1. Existence and Uniqueness

In this subsection we present a result which guarantees the existence and uniqueness of a solution to problem (1) when the mappings T and S satisfy a Wardowski's contractivity type condition.

Theorem 3.1. Let X be a nonempty set and let (Y, ρ) be a complete metric space. Assume that T, S:X → Y are two mappings satisfying the following conditions:

(i) T(X) is closed;

(ii) S(X) ⊆ T(X);

(iii) There exist τ > 0 and f ∈ ${F}$_k such that, for all x, y ∈ X,

$\begin{array}{cc}\rho (Sx,Sy)>0\Rightarrow \tau +f(\rho (Sx,Sy))\le f(\rho (Tx,Ty)).& (3)\end{array}$

Then, T and S have at least one coincidence point in X. If, moreover, T is one-to-one, then this coincidence point is unique.

Proof. Consider h : T(X) → 2^T(X) given by h(x): = S(T⁻¹x), where T⁻¹x: = {ξ ∈ X:T(ξ) = x}. Notice that h is single-valued. Indeed, if u, v ∈ h(x) with u ≠ v, then by definition we know that there exists ${\xi }_u,{\xi }_v\in T^{-1}x$ such that u = Sξ_u and v = Sξ_v. Since ρ(Sξ_u, Sξ_v) = ρ(u, v) > 0, from (3), we have that

$\tau +f(\rho (S{\xi }_u,S{\xi }_v))\le f(\rho (T{\xi }_u,T{\xi }_v))=f(\rho (x,x))=f(0),$

which is a contradiction, because f is not defined at 0.

Therefore, h : T(X) → T(X) is a single valued map from T(X) into itself. Furthermore, h verifies Wardowski's contractive condition [12], since if 0 < ρ(h(x), h(y)) = ρ(S(T⁻¹x), S(T⁻¹y)), then by (3) we have that

$\tau +f(\rho (S(T^{-1}x),S(T^{-1}y)))\le f(\rho (T(T^{-1}x),T(T^{-1}y))),$

that is, τ + f(ρ(h(x), h(y))) ≤ f(ρ(x, y)).

Bearing in mind that (Y, ρ) is complete and T(X) is closed, Wardowski's Theorem states that h has a unique fixed point y* ∈ T(X). Consider x* ∈ T⁻¹y*. Then, by definition, we have that Sx* = S(T⁻¹y*) = h(y*) = y* = Tx*, that is, x* is a coincidence point of T and S.

Now suppose that T is injective. If there exist x*, x′ ∈ X such that Sx* = Tx*, Tx′ = Sx′ and x* ≠ x′, then Sx* = Tx* ≠ Tx′ = Sx′ because T is injective. From (3), we obtain

$\tau +f(\rho (S{x}^{*},Sx\prime ))\le f(\rho (T{x}^{*},Tx\prime ))=f(\rho (S{x}^{*},Sx\prime )),$

i.e., τ ≤ 0 which is a contradiction.            □

Corollary 3.1. Let X be a nonempty set and (Y, ρ) be a complete metric space. Assume that T, S : X → Y are two mappings such that:

(a) T(X) is closed;

(b) S(X) ⊆ T(X);

(c) There exist τ > 0 such that, for all x, y ∈ X,

$\begin{array}{cc}\rho (Sx,Sy)>0\Rightarrow \rho (Sx,Sy)\le \frac{\rho (Tx,Ty)}{{(1+\tau \sqrt{\rho (Tx,Ty)})}^2}.& (4)\end{array}$

Then, T and S have at least one coincidence point in X. If, moreover, T is one to one, then this coincidence point is unique.

Proof. From (c) it follows that

$\sqrt{\rho (Sx,Sy)}\le \frac{\sqrt{\rho (Tx,Ty)}}{1+\tau \sqrt{\rho (Tx,Ty)}},$

that is,

$\frac{1+\tau \sqrt{\rho (Tx,Ty)}}{\sqrt{\rho (Tx,Ty)}}\le \frac{1}{\sqrt{\rho (Sx,Sy)}},$

therefore

$\tau +\frac{1}{\sqrt{\rho (Tx,Ty)}}\le \frac{1}{\sqrt{\rho (Sx,Sy)}}.$

The above inequality can be written as

$\tau -\frac{1}{\sqrt{\rho (Sx,Sy)}}\le -\frac{1}{\sqrt{\rho (Tx,Ty)}}.$

The last inequality means that T and S satisfy the conditions of Theorem 3.1 with respect to the function $f(t)=-\frac{1}{\sqrt{t}}$, which belongs to ${F}$_k for some $k\in (\frac{1}{2},1)$.            □

3.2. Picard-Juncgk's Iteration Process

In this subsection we present the results on the convergence for the Picard-Jungck scheme when the conditions of Theorem 3.1 are satisfied. Before giving our convergence result, we state the following lemma proved implicitly in the proof of Wardowski's Theorem [12].

Lemma 3.1. Let τ > 0 and f ∈ ${F}$_k with k ∈ (0, 1). If {γ_n}_n∈ℕ is a sequence of real non-negative numbers satisfying τ + f(γ_n+1) ≤ f(γ_n) for all n ∈ ℕ, then the series $\sum _{i=0}^{\infty }{\gamma }_i$ is convergent.

Theorem 3.2. Let X be a nonempty set and (Y, ρ) be a complete metric space. If T, S : X → Y satisfy the three conditions of Theorem 3.1 and T is one-to-one, then given x₁ ∈ X the iterative scheme Tx_n+1 = Sx_n satisfies that the sequences {Tx_n}_n∈ℕ and {Sx_n}_n∈ℕ converge to Tp = Sp, where p ∈ X is the unique coincidence point of T and S.

Proof. Notice that under these assumptions, Theorem 3.1 guarantees the existence and uniqueness of a coincidence point of T and S. Let x₁ ∈ X. It is worth pointing out that the sequence {x_n}_n∈ℕ, implicitly defined as

$\begin{array}{cc}T{x}_{n+1}=S{x}_n\text{for all }n\in \mathbb{N},& (5)\end{array}$

is well-defined, since S(X) ⊆ T(X). Furthermore, from the injectiveness of T, there exists T⁻¹:T(X) → X and, therefore, the sequence {x_n}_n∈ℕ can be explicitly defined by $x_{n+1}=T^{-1}(S{x}_n)$ for all n ∈ ℕ.

If there exists n₀ ∈ ℕ such that Sx_n0 = Sx_n0+1, then by (5) x_n0+1 is a coincidence point of T and S. But, in this case, we have that Tx_n0+2 = Sx_n0+1 = Tx_n0+1, which implies x_n0+2 = x_n0+1 because T is injective. Again applying (5), we deduce that Tx_n0+3 = Sx_n0+2 = Tx_n0+1. Bearing in mind the injectiveness of T, we get x_n0+3 = x_n0+1. Hence, {x_n}_n>n0 is a constant sequence.

Thus, we can assume that Sx_n ≠ Sx_n+1 for all n ∈ ℕ. For each n ∈ ℕ, we define γ_n: = ρ(Sx_n, Sx_n+1). Thus, γ_n>0 for all n ∈ ℕ. Moreover, from (3) and (5), τ+f(γ_n+1) ≤ f(γ_n) for all n ∈ ℕ. By Lemma 3.1, the series $\sum _{i=0}^{\infty }{\gamma }_i$ is convergent. Then, {Sx_n}_n∈ℕ is a Cauchy sequence, since for m ≥ n,

$\rho (S{x}_m,S{x}_n)\le {\gamma }_{m-1}+{\gamma }_{m-2}+\cdots +{\gamma }_n<{\displaystyle \sum _{i=n}^{\infty }{\gamma }_i}.$

Since T(X) is complete, there exists q ∈ T(X) such that Sx_n → q as n → ∞. By (5), we also deduce that Tx_n → q as n → ∞. Since q ∈ T(X), there exists p ∈ X such that q = Tp. Let us see that Tp = Sp.

Notice that there exists n₁ ∈ ℕ such that Sx_n ≠ Sp for all n ≥ n₁. Otherwise there exists a subsequence {Sx_nk}_nk∈ℕ such that Sx_nk = Sp for all n_k ∈ ℕ. In this case, Sp = Tp since Sx_n → Tp as n → ∞.

Therefore, we can assume that Sx_n ≠ Sp for all n ≥ n₁. By the contractive condition (3), for each n ≥ n₁,

$\tau +f(\rho (S{x}_n,Sp))\le f(\rho (T{x}_n,Tp)).$

Since τ > 0 and f is strictly increasing, we have that ρ(Sx_n, Sp) < ρ(Tx_n, Tp) for all n ≥ n₁. Taking limits and bearing in mind that Tx_n → Tp as n → ∞, we infer that Sx_n → Sp as n → ∞. Then, Tp = Sp.            □

We now state the convergence of the sequence {x_n}_n∈ℕ to the unique coincidence point of T and S.

Theorem 3.3. Let (X, d) and (Y, ρ) be two metric spaces, with Y being complete. Suppose that T, S : X → Y satisfy the three conditions of Theorem 3.1. If T is injective and T⁻¹ is continuous, then the sequence {x_n}_n∈ℕ, defined by $x_{n+1}=T^{-1}S{x}_n$ for each n ∈ ℕ, converges to the unique coincidence point of T and S.

Proof. Let p be the unique coincidence point of T and S, whose existence and uniqueness is guaranteed by Theorem 3.1. Fix x₁ ∈ X. By Theorem 3.2, we know that {Tx_n}_n∈ℕ and {Sx_n}_n∈ℕ converge to Tp = Sp. From the continuity of T⁻¹ we conclude that

$\underset{n\to \infty }{\lim }{x}_{n+1}=\underset{n\to \infty }{\lim }{T}^{-1}S{x}_n=T^{-1}(Tp)=p.$

            □

Notice that it is not easy to check the continuity of T⁻¹. However, one can give some metric type condition for T which implies the continuity of T⁻¹. In order to do this, we denote by ${G}$ the set of functions g:ℝ₊ → ℝ₊ such that, for any sequence {t_n}_n∈ℕ, $\underset{n\to \infty }{\lim }g(t_n)=0$ implies $\underset{n\to \infty }{\lim }{t}_n=0$. On one hand, it is easily seen that if g ∈ ${G}$ then g(t) > 0 for all t > 0. On the other hand, ${G}$ contains a large number of functions, because ${G}$ contains the set of all monotone nondecreasing real functions g:ℝ₊ → ℝ₊ such that g(t) = 0 if and only if t = 0, see [10, Lemma 2.2].

Corollary 3.2. Let (X, d) and (Y, ρ) be two metric spaces, with Y being complete. Suppose that T, S : X → Y satisfy the three conditions of Theorem 3.1. If there exists g ∈ ${G}$ such that

$\begin{array}{cc}g(d(x,y))\le \rho (Tx,Ty),forallx,y\in X,& (6)\end{array}$

then the sequence {x_n}_n∈ℕ, defined by $x_{n+1}=T^{-1}S{x}_n$ for each n ∈ ℕ, converges to the unique coincidence point of T and S.

Proof. It is sufficient to prove that T is one to one and T⁻¹ is continuous. Notice first that (6) implies that T is one to one. Indeed, if Tx = Ty then g(d(x, y)) = 0 which implies that d(x, y) = 0, since g ∈ ${G}$. Then, T⁻¹ : T(X) → X is well-defined. We now see that T⁻¹ is continuous. Let {u_n}_n∈ℕ ⊆ T(X) be a sequence converging to u ∈ T(X). From (6), we have g(d(T⁻¹v, T⁻¹w)) ≤ ρ(v, w) for all v, w ∈ T(X). Then,

$0\le \underset{n\to \infty }{\lim }g\left(d\left(T^{-1}{u}_n,T^{-1}u\right)\right)\le \underset{n\to \infty }{\lim }\rho (u_n,u)=0.$

Since g ∈ ${G}$, we deduce that $T^{-1}{u}_n\to T^{-1}u$ as n → ∞.            □

Remark 3.1. It is worth pointing out that the continuity of T⁻¹ does not imply that (6) holds: Just take T:ℝ₊ → ℝ₊ defined by $Tx=\sqrt{x}$.

Remark 3.2. Since the identity mapping is weakly compatible with respect to any mapping, from Corollary 3.2, we recapture Theorem 2.1.

Corollary 3.3. Let (X.d) and (Y, ρ) be two metric spaces, with Y being complete. Assume that T, S : X → Y are two mappings satisfying the conditions of Corollary 3.1 and, in addition, that there exists g ∈ ${G}$ such that T : X → Y satisfies inequality (Equation 6). Then the sequence {x_n}, defined by $x_{n+1}=T^{-1}(S{x}_n)$, converges to the unique coincidence point of T and S.

Proof. The proof of Corollary 3.1 shows that T and S satisfy the hypotheses of Corollary 3.2 with $f(t)=-\frac{1}{\sqrt{t}}$ and therefore we obtain the result.            □

3.3. Rate of Convergence

The idea given in Kohlenbach [13] allows us to introduce the concept of modulus of uniqueness for the coincidence problem as follows.

Definition 3.1. Let (X, d) and (Y, ρ) be two metric spaces and let T, S : X → Y be two mappings. A function ψ : (0, ∞) → (0, ∞) is said to be a modulus of uniqueness for the coincidence problem defined by T and S if, for any ε > 0, max{ρ(Tx, Sx), ρ(Ty, Sy)} < ψ(ε) implies that d(x, y) < ε.

Theorem 3.4. Let (X, d) and (Y, ρ) be two metric spaces. Suppose that T, S : X → Y satisfy the three conditions of Theorem 3.1 and also that there exists an increasing function g : (0, +∞) → (0, +∞) such that

$\begin{array}{cc}g\left(d(x,y)\right)\le \rho (Tx,Ty),forallx,y\in X.& (7)\end{array}$

If the function β : (0, +∞) → (0, +∞) defined by β(t): = t − f⁻¹(f(t)−τ) is increasing then $\psi :=\frac{1}{2}\beta ◦g$ is a modulus of uniqueness for the coincidence problem defined by T and S.

Proof. Let ε > 0 and x, y ∈ X such that max {ρ(Tx, Sx), ρ(Ty, Sy)} < ψ(ε). Notice that

$\begin{array}{l}\rho (Tx,Ty)\le \rho (Tx,Sx)+\rho (Sx,Sy)+\rho (Sy,Ty)<2\psi (\epsilon )\\ +f^{-1}(f(\rho (Tx,Ty))-\tau ).\hfill \end{array}$

Then, β(ρ(Tx, Ty)) < 2ψ(ε) = β(g(ε)). Since β is increasing, we get ρ(Tx, Ty) < g(ε). From (7), we deduce that d(x, y) < ε because g is increasing.            □

Remark 3.3. As a direct consequence of the above theorem, we can get a new result on generalized Ulam-Hyers stability of the coincidence problem (1).

Another consequence of Theorem 3.4 is the following result that states a rate of convergence for Picard-Juncgk's iteration process.

Theorem 3.5. Under the hypotheses of Theorem 3.4. Let {x_n}_n∈ℕ be the sequence defined by $x_{n+1}=T^{-1}S{x}_n$ for each n ∈ ℕ. Let p ∈ X be some coincidence point of T and S. Then, for all n ≥ Φ(ε), we have that d(x_n, p) < ε, where Φ : (0, +∞) → ℕ is given as

$\begin{array}{l}\Phi (\epsilon ):=\\ \{\begin{array}{cc}\lfloor \frac{f(\rho (S{x}_1,T{x}_1))-f(\psi (\epsilon ))}{\tau }\rfloor +2& if\psi (\epsilon )\le \rho (S{x}_1,T{x}_1),\\ 1& if\rho (S{x}_1,T{x}_1)<\psi (\epsilon ).\end{array}\end{array}$

Proof. Fix ε > 0. By Theorem 3.4, if we prove that ρ(Tx_n, Sx_n) < ψ(ε) for all n ≥ Φ(ε), then we are done, since in this case it is enough to take x = x_n and y = p.

Let us prove that ρ(Tx_n, Sx_n) < ψ(ε) for all n ≥ Φ(ε), i.e., ρ(Sx_n−1, Sx_n) < ψ(ε) for all n ≥ Φ(ε). From the proof of Theorem 3.2 we know that the sequence {γ_n}_n∈ℕ, defined by γ_n: = ρ(Sx_n, Sx_n+1), satisfies

$\begin{array}{cc}\tau +f({\gamma }_{n+1})\le f({\gamma }_n)\text{for all }n\in \mathbb{N}.& (8)\end{array}$

Since f is increasing and τ > 0, we have that {γ_n}_n∈ℕ is strictly decreasing.

If γ₁: = ρ(Sx₁, Tx₁) < ψ(ε), then γ_n < ψ(ε) for all n ≥ 1 = Φ(ε). Thus, we can assume that ψ(ε) ≤ γ₁.

We claim that γ_Φ(ε) < ψ(ε). By contradiction, suppose that ψ(ε) ≤ γ_Φ(ε). Using (8), we obtain that (Φ(ε)−1)τ + f(γ_Φ(ε)) ≤ f(γ₁). Bearing in mind that f is increasing, we deduce that (Φ(ε)−1)τ+f(ψ(ε)) ≤ f(γ₁), which contradicts the definition of Φ(ε). Therefore, γ_Φ(ε) < ψ(ε). Since {γ_n}_n∈ℕ is decreasing, we conclude that γ_n < ψ(ε) for all n ≥ Φ(ε).            □

Corollary 3.4. Let (X, d) and (Y, ρ) be two metric spaces. If T, S:X → Y satisfy the condition of Corollary 3.3, then the function $\psi (\epsilon )=\frac{1}{2}(\beta ◦g)(\epsilon )$, where $\beta (t)=t-\frac{1}{{(\tau +\frac{1}{\sqrt{t}})}^2}$, is a modulus of uniqueness for the coincidence problem defined by T and S.

Proof. In this case, the proof of Corollary 3.1 shows that $f(t)=-\frac{1}{\sqrt{t}}$, and then it is clear that $f^{-1}(t)=\frac{1}{t^2}$. The above facts imply that $\beta (t)=t-\frac{1}{{(\tau +\frac{1}{\sqrt{t}})}^2}$ and then its derivative is ${\beta }^{\prime }(t)=1-\frac{1}{{(1+\tau \sqrt{t})}^3}\ge 0$, which says that β is an increasing function. Finally, by Theorem 3.4, we infer that $\psi (ϵ)=\frac{1}{2}(\beta ◦g)(ϵ)$ is a modulus of uniqueness.            □

4. An Application to Differential Equations

We consider the following problem associated to a general differential equation of second order with homogeneous Dirichlet condition:

$\left(P\right)\{\begin{array}{l}u″(t)=G\left(t,u(t),u\prime (t)\right),\text{for }t\in [a,b]\hfill \\ u\left(a\right)=0,u\left(b\right)=0,\hfill \end{array}$

where G:[a, b] × ℝ × ℝ → ℝ is certain known function satisfying the following two general conditions:

(H₁) G is continuous in [a, b] × ℝ × ℝ;

(H₂) there exist τ, μ > 0 and f ∈ ${F}$_k, for some k ∈ (0, 1), such that

$\left|G(t,x_1,x_2)-G(t,y_1,y_2)\right|\le f^{-1}\left(f(\mu \underset{1=1,2}{\max }{\alpha }_i|x_i-y_i|)-\tau \right)$

for all t ∈ [0, 1], x_i, y_i ∈ ℝ, with i = 1, 2; where,

$0\le {\alpha }_1\le \frac{8}{\mu {(b-a)}^2}\text{and}0\le {\alpha }_2\le \frac{2}{\mu (b-a)}.$

Let Y = (${C}$[a, b], ||·||_∞) be the Banach space of the continuous functions u : [a, b] → ℝ, with its norm ||u||_∞: = max{|u(t) : a ≤ t ≤ b}. In the linear space ${C}$²[a, b]: = {u : [a, b] → ℝ : u″ ∈ ${C}$[a, b]} we consider the linear subspace X: = {u ∈ ${C}$²[a, b] : u(a) = u(b) = 0}. Notice that X endowed with the norm $\text{||}u{\text{||}}_{*}:=\max \{\text{||}u{\text{||}}_{\infty },\text{||}{u}^{\prime }{\text{||}}_{\infty },\text{||}{u}^{″}{\text{||}}_{\infty }\}$ is a Banach space.

In order to prove the existence and uniqueness of a solution of (P) in ${C}$²[a, b], we need the following result attributed to Tumura [14], see [15, p. 80].

Lemma 4.1. For any u ∈ X we have that $\text{||}u|{|}_{\infty }\le \frac{{(b-a)}^2}{8}||u^{″}|{|}_{\infty }$ and $\text{||}{u}^{\prime }{\text{||}}_{\infty }\le \frac{(b-a)}{2}\text{||}{u}^{″}{\text{||}}_{\infty }$. Moreover, the above inequalities are sharp, since they become equalities for the function u(t) = (t − a)(b − t).

Now we are able to state the main result of this section on the existence and uniqueness of a solution of (P).

Theorem 4.1. With the previous notation, suppose that: G : [a, b] × ℝ × ℝ → ℝ satisfies conditions (H₁) and (H₂). Then, problem (P) has a unique solution $u_s\in {{C}}^2\left[a,b\right]$.

Proof. We define T, S : X → Y as Tu(t) = u″(t) and Su = G(t, u(t), u′(t)). In order to obtain the existence and uniqueness of the solution to the problem (P), we will see that T and S satisfy the conditions of Theorem 3.1. Notice that T is onto. Indeed, given w ∈ Y it is enough to consider

$u(t):={\displaystyle {\int }_a^{t}v}(s)ds-\frac{t-a}{b-a}{\displaystyle {\int }_a^{b}v}(s)ds,\text{where }v(s):={\displaystyle {\int }_a^{s}w}(r)dr,$

since in this case u ∈ X and Tu = w. Thus, assumptions (i) and (ii) in Theorem 3.1 hold. Let us prove that T and S satisfy (iii). Assume that u, v ∈ X with ||u − v||_∞ ≠ 0. Then, there exists at least one t ∈ [a, b] such that u(t) ≠ v(t). Hence, by (H₂),

$\begin{array}{l}\left|Su(t)-Sv(t)\right|=\left|G(t,u(t),u\prime (t))-G(t,v(t),v\prime (t))\right|\\ \le f^{-1}(f(\mu \max \{{\alpha }_1\left|u(t)-v(t)\right|,\hfill \\ {\alpha }_2\left|u\prime (t)-v\prime (t)\right|\})-\tau )\hfill \\ \le f^{-1}(f(\mu \max \{{\alpha }_1{\Vert u(t)-v(t)\Vert }_{\infty },\hfill \\ {\alpha }_2{\Vert u\prime (t)-v\prime (t)\Vert }_{\infty }\})-\tau )\hfill \\ \le f^{-1}(f({\Vert u″-v″\Vert }_{\infty })-\tau ),\hfill \end{array}$

the last inequality is obtained from Lemma 4.1 and because f is increasing. Thus, $\text{||}Su-Sv{\text{||}}_{\infty }\le f^{-1}(f(\text{||}Tu-Tv{\text{||}}_{\infty })-\tau )$, that is, T and S satisfy (iii). From Theorem 3.1, T and S have a unique coincidence point in X, i.e., problem (P) has a unique solution $u_s\in {{C}}^2\left[a,b\right]$.

Remark 4.1. Under the conditions of Theorem 4.1, applying Lemma 4.1 we obtain that $\text{||}u{\text{||}}_{*}\le M\text{||}{u}^{″}{\text{||}}_{\infty }$, where

$M:=\max \left\{\frac{{(b-a)}^2}{8},\frac{b-a}{2},1\right\}.$

Then

(a) If we define g(t) = t/M, it is clear that T satisfies inequalities (Equations 6, 7). Therefore, by Corollary 3.2, we infer that for each u₁ ∈ X, the sequence {u_n}_n∈ℕ defined by

$\begin{array}{cc}\begin{array}{l}{u}_{n+1}(t):={\displaystyle {\int }_a^t\left({\displaystyle {\int }_a^s{G}_n}(r)dr\right)}ds\\ -\frac{t-a}{b-a}{\displaystyle {\int }_a^b\left({\displaystyle {\int }_a^s{G}_n}(r)dr\right)}ds,\end{array}& (9)\end{array}$

where $G_n(r):=Gr,u_n(r),u_n^{\prime }(r)$, converges to u_s,

(b) If the function β : (0, ∞) → ℝ, defined by β(t): = t − f⁻¹ (f(t) − τ), is increasing, Theorem 3.5 yields that for any ε > 0, ||u_n − u_s||_* < ε for all n ≥ Φ(ε), where

$\begin{array}{l}\Phi (\epsilon ):=\\ \{\begin{array}{l}\lfloor \frac{f\left({\Vert u_2^{″}-u_1^{″}\Vert }_{\infty }\right)-f(\beta (g(\epsilon ))/2)}{\tau }\rfloor +2,if\beta (g(\epsilon ))\le 2{\Vert u_2^{″}-u_1^{″}\Vert }_{\infty },\hfill \\ 1,if2{\Vert u_2^{″}-u_1^{″}\Vert }_{\infty }<\beta (g(\epsilon )),\hfill \end{array}& (10)\end{array}$

which means that Φ given by (10) is a rate of convergence for {u_n} to u_s.

4.1. A Particular Case

Let G : [0, 1] × ℝ × ℝ → ℝ be a continuous function such that for every t ∈ [0, 1], and for all x, y ∈ ℝ the following inequality holds for some τ > 0,

$\begin{array}{cc}\left|G(t,x,y)-G(t,u,v)\right|\le \frac{\max \left\{|x-u|,|y-v|\right\}}{(1+\tau \sqrt{\max \left\{|x-u|,|y-v|\right\}{)}^2}}.& (11)\end{array}$

Let us check that G satisfies condition (H₂). Indeed, consider the function f:(0, ∞) → (−∞, 0) defined by $f(t)=-\frac{1}{\sqrt{t}}.$ It is clear that f⁻¹ : (−∞, 0) → (0, ∞) is given by $f^{-1}(s)=\frac{1}{s^2}.$ Therefore, taking μ = α₁ = α₂ = 1 we have:

$\begin{array}{l}{f}^{-1}(f(\max \left\{\right|x-u|,|y-v\left|\right\})-\tau ))\\ =\frac{1}{{(f(\max \left\{\right|x-u|,|y-v\left|\right\})-\tau )}^2}\hfill \\ =\frac{\max \left\{\right|x-u|,|y-v\left|\right\}}{{(1+\tau \sqrt{\max \left\{\right|x-u|,|y-v\left|\right\}})}^2},\hfill \end{array}$

which means that G satisfies condition (H₂).

Example 4.1. The second order differential equation with homogeneous Dirichlet condition

$\begin{array}{cc}\{\begin{array}{l}u″(t)=e^t+\frac{|u(t)|}{{(\sqrt{2}+\sqrt{2|u(t)|})}^2}+\frac{|u\prime (t)|}{{(\sqrt{2}+\sqrt{2|u\prime (t)|})}^2},fort\in [0,1],\\ u(0)=0,u(1)=0,\end{array}& (12)\end{array}$

has a unique classical solution.

To see that Equation (12) has a unique classical solution it is enough to show that the conditions of Theorem 4.1 are satisfied. Since Equation (12) can be rewritten as

$\begin{array}{cc}\{\begin{array}{l}u″(t)=G(t,u(t),u\prime (t)),fort\in [0,1],\hfill \\ u(0)=0,u(1)=0,\hfill \end{array}& (13)\end{array}$

where G : [0, 1] × ℝ × ℝ → ℝ is defined by

$G(t,x,y)=e^t+\frac{1}{2}[\frac{\left|x\right|}{{(1+\sqrt{\left|x\right|})}^2}+\frac{\left|y\right|}{{(1+\sqrt{\left|y\right|})}^2}],$

we are going to prove that G satisfies inequality (Equation 11). To do this, we notice first that the following elementary properties hold:

(1) the function φ : [0, ∞) → [0, ∞), $\phi (t)=\frac{t}{{(1+\sqrt{t})}^2}$, is increasing since ${\phi }^{\prime }(t)=\frac{1}{{(1+\sqrt{t})}^3}>0,$

(2) φ is concave since ${\phi }^{″}(t)=\frac{-3}{2\sqrt{t}{(1+\sqrt{t})}^4}<0,$

(3) since φ(0) = 0 and φ is concave, then it is sub-additive, that is φ(t + s) ≤ φ(t) + φ(s).

Since

$\begin{array}{l}\left|G(t,x,y)-G(t,u,v)\right|\le \frac{1}{2}\left|\frac{\left|x\right|}{{(1+\sqrt{\left|x\right|})}^2}-\frac{\left|u\right|}{{(1+\sqrt{\left|u\right|})}^2}\right|\\ +\frac{1}{2}\left|\frac{\left|y\right|}{{(1+\sqrt{\left|y\right|})}^2}-\frac{\left|v\right|}{{(1+\sqrt{\left|v\right|})}^2}\right|\hfill \end{array}$

With the above three properties, the above inequality can be written as follows

$\begin{array}{l}\left|G(t,x,y)-G(t,u,v)\right|\le \frac{1}{2}\left|\phi (|x|)-\phi (|u|)\right|+\frac{1}{2}\left|\phi (|y|)-\phi (|u|)\right|\\ \le \frac{1}{2}\left|\phi (|x|-|u|)\right|+\frac{1}{2}\left|\phi (|y|-|v|)\right|\hfill \\ \le \frac{1}{2}\phi (|x-u|)+\frac{1}{2}\phi (|y-v|)\hfill \\ \le \phi (\max \{|x-u|,|y-v|\})\hfill \\ =\frac{\max \left\{\right|x-u|,|y-v\left|\right\}}{(1+\sqrt{\max \left\{\right|x-u|,|y-v\left|\right\}{)}^2}},\hfill \end{array}$

which means that the conditions of Theorem 4.1 are satisfied and therefore Equation (12) admits a unique classical solution.

Finally, let us give, by using expression (10), a rate of convergence for the iterative scheme given in Equation (9) concerning Equation (12). To apply Theorem 3.5, first we have to notice that the following facts hold:

1. f : (0, +∞) → (−∞, 0) is given by $f(t)=-\frac{1}{\sqrt{t}}$,

2. f⁻¹ : (−∞, 0) → (0, +∞) is $f^{-1}(s)=\frac{1}{s^2}$.

3. g:[0, ∞) → [0, ∞) is given by g(t) = t,

4. τ = 1,

5. $\beta (t)=t-\frac{t}{{(1+\sqrt{t})}^2},$

6. $\psi (ϵ)=\frac{1}{2}\beta (ϵ).$

In Figure 1, we use the above facts and expression (Equation 10) to compute the number of iterations that we have to do to obtain an error less than ϵ = 10^−k for k = 1, ⋯ , 6 and taking as starting points u₁ = 0 and $u_1=t^2-t$ respectively.

FIGURE 1

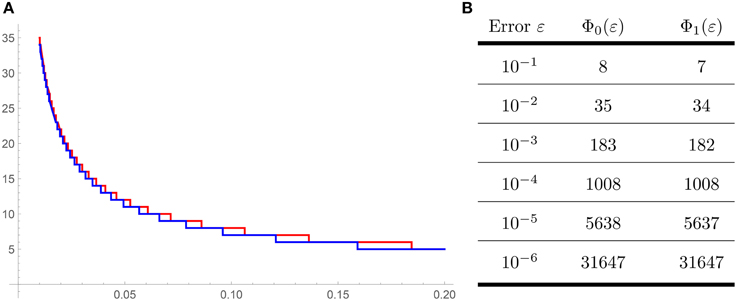
Figure 1. (A) Red line is the ratio taking as a starting point u₁ = 0 and blue line is the ratio for the starting point $u_1=t^2-t$. (B) (The ratio of convergence Φ₀ corresponds to u₁ = 0, while Φ₂ corresponds to $u_1=t^2-t$).

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

Part of this work was carried out while the first author was visiting the Department of Mathematical Analysis of the University of Valencia.

The research of the first author has been partially supported by MTM 2012-34847-C02-01 and P08-FQM-03453. The second author has been partially supported by MTM 2012-34847-C02-02.

References

1. Garcia-Falset J, Mleşnitţe O. Coincidence problems for generalized contractions. Appl Anal Discrete Math. (2014) 8:1–15. doi: 10.2298/AADM131031021F

CrossRef Full Text | Google Scholar

2. Garcia-Falset J, Muñiz-Pérez O, Sadarangani K. Coincidence problems under contractive type conditions. Fixed Point Theory. (in press).

3. Goebel K. A coincidence Theorem. Bull L'Acad Pol Sci. (1968) 16:733–5.

4. Machuca R. A coincidence problem. Amer Math Month (1967) 74:469. doi: 10.2307/2314896

CrossRef Full Text

5. Mleşnite O. Existence and Ulam-Hyers stability results for coincidence problems. J Nonlinear Sci Appl. (2013) 6:108–16.

Google Scholar

6. Berinde V. Common fixed points of noncommuting discontinuous weakly contractive mappings in cone metric spaces. Taiwan J Math. (2010) 14:1763–76.

Google Scholar

7. Cakić N. Coincidence and common fixed point theorems for (φ, ϕ) weakly contractive mappings in generalized metric spaces. Filomat (2013) 27:1415–23. doi: 10.2298/FIL1308415C

PubMed Abstract | CrossRef Full Text | Google Scholar

8. Jungck G. Commuting mappings and fixed points. Am Math Month. (1976) 83:261–3. doi: 10.2307/2318216

CrossRef Full Text | Google Scholar

9. Abbas M, Jungck G. Common fixed point results for noncommuting mappings without continuity in cone metric spaces. J Math Anal Appl. (2008) 341:416–20. doi: 10.1016/j.jmaa.2007.09.070

CrossRef Full Text | Google Scholar

10. Ariza-Ruiz D, Garcia-Falset J. Iterative approximation to a coincidence point of two mappings. Appl Math Comp. (2015) 259:762–76. doi: 10.1016/j.amc.2015.03.007

CrossRef Full Text | Google Scholar

11. Olaleru JO, Akewe H. On Multistep Iterative scheme for approximating the common fixed point of contractive-like operators. Inter J Math Math Sci. (2010) 2010:530964. doi: 10.1155/2010/530964

CrossRef Full Text | Google Scholar

12. Wardowski D. Fixed points of a new type of contractive mappings in complete metric spaces. Fixed Point Theory Appl. (2012) 2012:1–6. doi: 10.1186/1687-1812-2012-94

CrossRef Full Text | Google Scholar

13. Kohlenbach U. Applied Proof Theory. Proof Interpretations and their Use in Mathematics. Berlin; Heidelberg: Springer Monographs in Mathematics (2008).

Google Scholar

14. Tumura M, Kôkai zyôbibunhôteisiki ni tuite. Kansû Hôteisiki (1941) 30:20-35.

15. Mitrinović DS, Pečarić JE, Fink AM. Inequalities Involving Functions and their Integrals and Derivatives. Dordrecht: Kluwer Academic Publishers Group (1991). doi: 10.1007/978-94-011-3562-7

CrossRef Full Text | Google Scholar